Utilisateur:RudeWolf/traduction/Groupes (mathématiques)

Les réagencements d'un Rubik's Cube forment un groupe, nommé le groupe du Rubik's Cube.

En mathématiques, un groupe désigne un ensemble d'éléments, muni d'une loi de composition interne qui permet de combiner deux éléments quelconques en un troisième. Pour former un groupe, cet ensemble et cette opération doivent satisfaire quelques conditions, des « axiomes »: l'associativité, l'existence d'un élément neutre, et l'existence, pour tout élément, d'un inverse. Ces conditions sont naturelles et se retrouvent dans beaucoup de constructions mathématiques, comme les systèmes numériques: par exemple l'ensemble Z des entiers relatifs, muni de l'opération d'addition « + », forme un groupe. Toutefois l'énoncé des axiomes des groupes ne s'intéresse pas à la nature concrète de la loi du groupe, ni des éléments sur lesquels elle opère. Cela permet de manipuler des objets variés, d'origines très diverses, dans un cadre unifié, tout en gardant la flexibilité et les habitudes qui nous viennent du calcul algébrique. L'ubiquité des groupes, en mathématiques et en dehors, en font thème central des mathématiques modernes.

Les groupes tutoient étroitement la notion de symétrie. On traduit les propriétés de symétrie d'un objet géométrique dans son groupe de symétrie: il s'agit de l'ensemble des transformations qui laisse un objet globalement inchangé, la loi composant deux transformations en une troisième obtenue en faisant agir l'une avant l'autre. Les groupes de symétrie, et en particulier les groupes continus de transformations introduits par S. Lie, jouent un rôle incontournable dans de nombreuses disciplines, comme les symétries internes, comme le spin, en physique des particules, les transformations de Lorentz en relativité restreinte, les groupes cristallographiques en la chimie moléculaire, etc.

La notion abstraite de groupe tire son origine de la théorie des équations polynomiales des années 1830, grâce à l'étude par Évariste Galois de permutations des racines d'une équation. La notion générale de groupe ne fut fermement établie qu'autour de 1870, après des apports venus de la théorie des nombres et de la géométrie. Depuis, l'étude des groupes en tant que tels est devenue une discipline propre, et toujours très active, des mathématiques, la théorie des groupes. Pour étudier les groupes, les mathématiques ont développé des notions variées pour décrire un groupe donné en termes de constituants plus simples et mieux compris, comme la notion de sous-groupe, celle de groupe quotient, de groupe simple. Une autre stratégie d'étude d'un groupe consiste, un groupe étant donné, de trouver une manière concrète de décrire ce groupe comme transformations d'un espace ou d'une structure bien connu, ce qui conduit à la notion de représentation. Un théorie particulièrement riche s'est développée pour les groupes finis, le point d'orgue en étant la classification des groupes finis simples, dont la démonstration monumentale fut achevée en 1983.

Modèle:TOClimit

Définition et Illustration

Un Premier example: les entiers

Un des exemple les plus familier de groupe est donné par l'ensemble Z des entiers relatifs:

..., −4, −3, −2, −1, 0, 1, 2, 3, 4, ...

Les propriétés formelles ci-dessous de l'addition sert de modèle pour les axiomes de la théorie des groupes qui apparaissent dans la définition plus bas.

• Pour deux entiers a et b, la somme a + b est aussi un entier. En d'autres mots, l'opération qui consiste à ajouter un entier à un autre ne produit jamais de résultat qui ne soit un entier. On dit aussi que l'ensemble des entiers est clos par addition.
• Chaque fois que l'on se donne trois entiers a, b et c, on a l'égalité suivante.

(a + b) + c = a + (b + c)

Autrement dit ajouter d'abord b à a, puis c à la somme obtenue donne le même résultat final que si l'on avait ajouté la somme de b et c à a. C'est cette propriété que l'on nomme l'associativité de l'addition.

• Il y a un entier particulier, noté 0. Il a cette particularité que peu importe l'entier a, on a

0 + a = a + 0 = a.

Zero est dit élément neutre pour l'addition: l'ajouter à un entier ne produit aucun effet.

• Pour tout entier a, il existe un autre entier b tel que

a + b = b + a = 0.

Lorsque a est fixé, il n'y a qu'un seul entier b comme ci-dessus. On dit que b est l'opposé de l'entier a. Ajouter b a l'effet inverse à celui obtenu en ajoutant a. On utilise la notation -a pour désigner l'opposé de a.

Définition

L'ensemble de tous les entiers, muni de l'addition forme un objet mathématique qui appartient à une grande famille d'objets possédant une structure analogue. Afin d'étudier abstraitement ces objets sans avoir besoin de manipuler chacun séparément, on a développé la définition ci-dessous. Elle permet de traiter l'exemple ci-dessus aussi bien que beaucoup d'autres, comme les groupes de symétrie que nous détaillerons plus bas. On définit un groupe en se donnant un ensemble, disons G, et une loi de composition interne sur cet ensemble, notons-la « • »: chaque fois que l'on se donne un premier élément a de G, puis un deuxième élément b de G, la loi nous dit comment en produire un troisième élément, toujours dans G, qui dépend invariablement de a et b, et qu'on désigne par a•b. Le symbole « • » est un symbole générique, qui, pourra selon les cas remplacer le symbole + de l'addition, × de la multiplication, ou encore $\circ$ de la composition. Pour que G, muni de la loi •, forme un groupe, on demande que soient satisfaites les conditions suivantes, dites axiomes de la théorie des groupes.

1.	Clôture.	Pour tous a et b dans G, le résultat produit par l'opération a•b est encore un élément de G.
2.	Associativité.	Pour tous a, b et c dans G, on a l'égalité (a • b) • c = a • (b • c).
3.	Élément neutre.	Il existe un élément e dans G tel que, pour tout élément a de G, l'égalité e • a = a • e = a soit vérifiée. On dit alors que e est un élément neutre.
4.	Inverse d'un élément.	Pour tout a dans G, il existe un élément b dans G tel que a•b = b • a = e, où e désigne un élément neutre.

Lorsque les axiomes ci-dessus sont vérifiés, on dit que (G,•) est un groupe et que • est une loi de groupe. Pour éviter toute ambigüité, il convient de désigner par (G,•) la structure considérée. Toutefois il arrive souvent, le contexte aidant, qu'on ne mentionne que l'ensemble G. Par exemple on dit parfois que « l'ensemble Z des entiers relatifs constitue un groupe ». Cela constitue un abus de langage, la loi •, ici l'addition +, étant sous-entendue.

Dans un groupe, l'ordre dans lequel les éléments d'un groupe sont combinés importe souvent, contrairement à l'exemple de l'addition sur les nombres entiers. Cela signifie que combiner a par b ne renvoie pas le même résultat que la combinaison de b par a; en termes algébriques, l'équation a • b = b • a n'est pas toujours vérifiée. En effet, bien que cette équation soit toujours vérifiée si l'on remplace a et b par des entiers et • par le symbole + de l'addition entre entiers, ce n'est déjà plus forcément le cas pour les groupes de symétrie plus bas. On appelle cette propriété la commutativité de la loi. Un groupe (G,•) qui, outre les axiomes 1. à 4. ci-dessus, vérifie aussi l'axiome de commutativité

5.

Commutativité.

Pour tous a et b dans G, l'égalité a•b = b • a est vérifiée.

est dit groupe abélien en l'honneur de Niels Abel.

Un Deuxième exemple: un groupe de symétrie

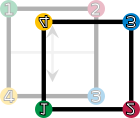
Les symétries du carré, plus précisément les réflections et les rotations qui laisse le carré globalement inchangé, constitue un groupe, pour la loi de composition interne qui consiste à envoyer deux transformations sur la transformation composée. C'est un groupe noté D₄. Il comprend les symétries suivantes.

id (ne rien bouger)	r1 (rotation d'un quart de tour vers la droite)	r2 (rotation d'un demi-tour)	r3 (rotation de trois quarts de tour vers la droite)
fv (retournement autour d'une verticale)	fh (retournement autour d'une horizontale)	fd (retournement autour de la diagonale ascendante)	fc (retournement autour de la diagonale descendante).
Les différents éléments du groupe de symétrie du carré (D4). Les sommets ont étés colorés et numérotés afin de visualiser les opérations.

• la transformation identique qui laisse tout tel quel, notée id ;
• les rotations d'un quart, d'un demi, et de trois quarts de tours vers la droite, notées r₁, r₂ et r₃ respectivement ;
• les retournements autours des axes vertical, horizontal (f_h et f_v), ou des deux diagonales (f_d et f_c).

---

On peut toujours composer deux symétries a et b, en les appliquant l'une après l'autre. On notera :b • a l'opération résultant à appliquer d'abord a puis b. La notation inversée, de droite à gauche, vient de la composition des fonctions. Ainsi si on note a(s) le sommet obtenu en appliquant a au sommet s, la notation b•a(s) reprend l'ordre d'écriture que l'on retrouve en considérant b(a(s)): à chaque fois on applique a avant b.

La table de la loi de groupe, affichée à droite, résume toute les compositions possibles de deux transformations. Par exemple, si l'on applique la rotation r₃ de trois quarts de tours sur la droite, puis le retournement f_h par rapport à l'axe horizontal, le résultat est le même que celui produit par au retournement f_d par rapport à la diagonale ascendante. Ceci se lit (en bleu) sur la table, et se résume en utilisant la notation • par

f_h • r₃ = f_d.

Group table of D₄

•	id	r1	r2	r3	fv	fh	fd	fc
id	id	r1	r2	r3	fv	fh	fd	fc
r1	r1	r2	r3	id	fc	fd	fv	fh
r2	r2	r3	id	r1	fh	fv	fc	fd
r3	r3	id	r1	r2	fd	fc	fh	fv
fv	fv	fd	fh	fc	id	r2	r1	r3
fh	fh	fc	fv	fd	r2	id	r3	r1
fd	fd	fh	fc	fv	r3	r1	id	r2
fc	fc	fv	fd	fh	r1	r3	r2	id
Les éléments id, r1, r2 et r3 forment un sous-groupe, représenté par la partie rouge de la table. Une classe à gauche et une classe à droite par ce sous-groupe sont indiquées en vert (dans la dernière ligne) et jaune (dernière colonne) respectivement.

Dans le cas de cet ensemble de symétries, et pour la loi de composition indiquée, les axiomes de la théorie des groupes s'interprètent comme suit:

1. La clôture demande que la composition b • a deux symétriesa et b résulte encore en une symétrie. Cela se vérifie déjà dans l'exemple de composition donné plus haut

   r₃ • f_h = f_c,

   i.e. rotating 270° right after flipping horizontally equals flipping along the counter-diagonal (f_c). Indeed every other combination of two symmetries still gives a symmetry, as can be checked using the group table.
2. La contrainte d'associativité concerne la composition de plus que deux symétries : given three elements a, b et c de D₄, there are two possible ways of computing "a then b then c". The requirement

   (a • b) • c = a • (b • c)

   means that the composition of the three elements is independent of the priority of the operations, i.e. composing first a after b et c to the result a • b thereof amounts to performing a after the composition of b et c. For example, (f_d • f_v) • r₂ = f_d • (f_v • r₂) can be checked using the group table at the right

   (fd • fv) • r2	=	r3 • r2	=	r1, which equals
   fd • (fv • r2)	=	fd • fh	=	r1.

3. The identity element is the symmetry id leaving everything unchanged: for any symmetry a, performing id after a (or a after id) equals a, in symbolic form,

   id • a = a,

   a • id = a.

4. An inverse element undoes the transformation of some other element. Every symmetry can be undone : each of transformations — identity id, the flips f_h, f_v, f_d, f_c et the 180° rotation r₂ — is its own inverse, because performing each one twice brings the square back to its original orientation. The rotations r₃ et r₁ are each other's inverse, because rotating one way et then by the same angle the other way leaves the square unchanged. In symbols,

   f_h • f_h = id,

   r₃ • r₁ = r₁ • r₃ = id.

In contrast to the group of integers above, where the order of the operation is irrelevant, it does matter in D₄: f_h • r₁ = f_c but r₁ • f_h = f_d. In other words, D₄ is not abelian, which makes the group structure more difficult than the integers introduced first.

History

La notion moderne de groupe s'est élaborée sous l'impulsion de différents domaines des mathématiques

The modern concept of an abstract group developed out of several fields of mathematics. Un des premier problèmes ayant motivé l'introduction de groupes est la recherche de solutions par radicaux pour des équations polynomiales de degré au moins 5. Au XIX^e siècle, Évariste Galois trouva, se basant sur des travaux antérieurs de Paolo Ruffini et Joseph-Louis Lagrange, un critère permettant de décider la sovalbilité d'une équation polynomiale donnée. Ce critère s'exprime en terme d'un groupe de symétrie de l'équation, formé de certaines permutations des racines. Les travaux de Galois ne furent pas compris, ou reconnus, par ses contemporains. Ses écrits, parfois oubliés ou égarés, n'accédèrent à la publication qu'après sa mort. Des groupes de permutations plus généraux sont ensuite apparus dans les travaux d'Augustin Louis Cauchy. C'est Arthur Cayley, dans son On the theory of groups, as depending on the symbolic equation θⁿ = 1 (1854) qui aurait donné la première définition abstraite d'un groupe fini en général.

La géométrie fut une seconde source de questions pour lesquelles la notion de groupe paru pertinente. Ce fut particulièrement le cas du Programme d'Erlangen de Felix Klein où elle fut d'usage systématique. Klein cherchait un principe organisationnel pour appréhender les nouveaux types d'espaces géométriques qui émergeaient alors, comme les espaces non euclidien, hyperboliques, projectifs... Peu après, en 1884, Sophus Lie introduisit l'étude des groupes à paramètres, qui combinent à la fois une structure de groupe et une structure d'espace géométrique.

Un autre domaine ayant contribué à la théorie des groupes est la théorie des nombres. Certains roupes abéliens apparaissaient déjà implicitement dans les travaux de Gauß sur la théorie des nombres, en particulier son célèbre Disquisitiones Arithmeticae (1798). Leopold Kronecker fit un usage plus explicite des groupes. Lors de sa tentative de démonstration du Théorème de Fermat, en 1847, Ernst Kummer manipula le groupe de classes, qui décrit les propriétés de factorisation en nombres premiers dans un système de nombres.

C'est Camille Jordan qui commença l'élaboration d'une théorie unifiant ces groupes, malgrés leurs aspects très divers, dans son Traité des substitutions et des équations algébriques (1870). Walther von Dyck (1882) formula le premier la définition actuelle de la notion de groupe. Au XX^e siècle, les groupes manifestèrent leur ubiquité grâce aux travaux fondateurs de Ferdinand Georg Frobenius et William Burnside sur la théorie des représentation des groupes finis, Richard Brauer et Issai Schur. La théorie des groupes de Lie, et dans un cadre plus général des groupes localement compacts fut énormément développée par, entre autres, par Hermann Weyl, Élie Cartan. Sa contre-partie algébrique, la théorie des groupes algébriques, fut d'abord délimité par Claude Chevalley (vers la foin des années 1930) puis ensuite par les travaux fondateurs d'Armand Borel et Jacques Tits.

La classification des groupes finis simples fut achevée en 1982, suite à un programme monumental, qui est le fruit d'une collaboration de nombreux mathématiciens, fondée 20 ans plus tôt sous l'impulsion de théoriciens des groupes comme Daniel Gorenstein, John G. Thompson et Walter Feit. Les travaux produits excèdent de très loin tous les projets antérieurs en mathématiques par plusieurs aspects: le nombre de collaborateurs, la longueur des preuves. La vérification et la validation des preuves furent l'origine de controverses. Aujourd'hui encore la simplification de ces preuves est un sujet de recherche actif. De nos jours, tout autant que jadis, la théorie des groupes est une branche très active qui influence très fortement de nombreux domaines de recherche.{cref|a}}

Simple consequences of the group axioms

Basic facts about all groups that can be obtained directly from the group axioms are commonly subsumed under elementary group theory. For example, repeated applications of the associativity axiom show that the unambiguity of

a • b • c = (a • b) • c = a • (b • c)

generalizes to more than three factors. Because this implies that parentheses can be inserted anywhere within such a series of terms, parentheses are usually omitted.

The axioms may be weakened to assert only the existence of a left identity et left inverses. Both can be shown to be actually two-sided, so the resulting definition is equivalent to the one given above.

Uniqueness of identity element et inverses

Two important consequences of the group axioms are the uniqueness of the identity element et the uniqueness of inverse elements. There can be only one identity element in a group, et each element in a group has exactly one inverse element. Thus, it is customary to speak of the identity, et the inverse of an element.

To prove the uniqueness of an inverse element of a, suppose that a has two inverses, denoted l et r. Then

l	=	l • e		as e is the identity element
	=	l • (a • r)		because r is an inverse of a, so e = a • r
	=	(l • a) • r		by associativity, which allows to rearrange the parentheses
	=	e • r		since l is an inverse of a, i.e. l • a = e
	=	r		for e is the identity element

Hence the two extremal terms l et r are connected by a chain of equalities, so they agree. In other words there is only one inverse element of a.

Division

In groups, it is possible to perform division : given elements a et b of the group G, there is exactly one solution x in G to the equation x • a = b. In fact, right multiplication of the equation by a⁻¹ gives the solution x = x • a • a⁻¹ = b • a⁻¹. Similarly there is exactly one solution y in G to the equation a • y = b, namely y = a⁻¹ • b. In general, x et y need not agree.

Basic concepts

The following sections use mathematical symbols such as X = {x, y, z} to denote a set X containing elements x, y et z, or alternatively x ∈ X to restate that x is an element of X. The notation f : X → Y means f is a function assigning to every element of X an element of Y.

Modèle:See To understand groups beyond the level of mere symbolic manipulations as above, more structural concepts have to be employed. There is a conceptual principle underlying all of the following notions: to take advantage of the structure offered by groups (which for example sets—being "structureless"—don't have) constructions related to groups have to be compatible with the group operation. This compatibility manifests itself in the following notions in various ways. For example, groups can be related to each other via functions called group homomorphisms. By the mentioned principle, they are required to respect the group structures in a precise sense. The structure of groups can also be understood by breaking them into pieces called subgroups et quotient groups. The principle of "preserving structures"—a recurring topic in mathematics throughout—is an instance of working in a category, in this case the category of groups.

Group homomorphisms

Group homomorphisms are functions that preserve group structure. A function a: G → H between two groups is a homomorphism if the equation

a(g • k) = a(g) • a(k).

holds for all elements g, k in G, i.e. the result is the same when performing the group operation after or before applying the map a. This requirement ensures that a(1_G) = 1_H, et also a(g)⁻¹ = a(g⁻¹) for all g in G. Thus a group homomorphism respects all the structure of G provided by the group axioms.

Two groups G et H are called isomorphic if there exist group homomorphisms a: G → H et b: H → G, such that applying the two functions one after another (in each of the two possible orders) equal the identity function of G et H, respectively. That is, a(b(h)) = h et b(a(g)) = g for any g in G et h in H. From an abstract point of view, isomorphic groups carry the same information. For example, proving that g • g = 1 for some element g of G is equivalent to proving that a(g) • a(g) = 1, because applying a to the first equality yields the second, et applying b to the second gives back the first.

Subgroups

Informally, a subgroup is a group H contained within a bigger one, G. Concretely, the identity element of G is contained in H, et whenever h₁ et h₂ are in H, then so are h₁ • h₂ et h₁⁻¹, so the elements of H, equipped with the group operation on G restricted to H, form indeed a group.

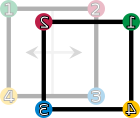
In the example above, the identity et the rotations constitute a subgroup R = {id, r₁, r₂, r₃}, highlighted in red in the group table above : any two rotations composed are still a rotation, et a rotation can be undone by (i.e. is inverse to) the rotation in the opposite direction. The subgroup test is a necessary and sufficient condition for a subset H of a group G to be a subgroup: it is sufficient to check that g⁻¹h ∈ H for all elements g, h ∈ H. Knowing the subgroups is important in understanding the group as a whole.

Given any subset S of a group G, the subgroup generated by S consists of products of elements of S et their inverses. It is the smallest subgroup of G containing S. In the introductory example above, the subgroup generated by r₂ et f_v consists of these two elements, the identity element id et f_h = f_v • r₂. Again, this is a subgroup, because combining any two of these four elements or their inverses (which are, in this particular case, these same elements) yields an element of this subgroup.

Cosets

In many situations it is desirable to consider two group elements the same if they differ by an element of a given subgroup. For example, in D₄ above, once a flip is performed, the square never gets back to the r₂ configuration by just applying the rotation operations (et no further flips), i.e. the rotation operations are irrelevant to the question whether a flip has been performed. Cosets are used to formalize this insight : a subgroup H defines left et right cosets, which can be thought of as translations of H by arbitrary group elements g. In symbolic terms, the left and right coset of H containing g are

gH = {gh, h ∈ H} and Hg = {hg, h ∈ H}, respectively.

The cosets of any subgroup H form a partition of G; that is, two left cosets are either equal or have an empty intersection. The first case g₁H = g₂H happens precisely when g₁⁻¹g₂ ∈ H, i.e. if the two elements differ by an element of H. Similar considerations apply to the right cosets of H. The left and right cosets of H may or may not be equal. If they are, i.e. for all g in G, gH = Hg, then H is said to be a normal subgroup. One may then simply refer to N as the set of cosets.

In D₄, the introductory symmetry group, the left cosets gR of the subgroup R consisting of the rotations are either equal to R, if g is an element of R itself, or otherwise equal to U = f_vR = {f_v, f_d, f_h, f_c} (highlighted in green). The subgroup R is also normal, because f_vR = U = Rf_v and similarly for any element other than f_v.

Quotient groups

In addition to disregarding the internal structure of a subgroup by considering its cosets, it is desirable to endow this coarser entity with a group law called quotient group or factor group. For this to be possible, the subgroup has to be normal. Given any normal subgroup N, the quotient group is defined by

G / N = {gN, g ∈ G}, "G modulo N"

This set inherits a group operation (sometimes called coset multiplication, or coset addition) from the original group G: (gN) • (hN) = (gh)N for all g and h in G. This definition is motivated by the idea (itself an instance of general structural considerations outlined above) that the map G → G / N that associates to any element g its coset gN be a group homomorphism, or by general abstract considerations called universal properties. The coset eN = N serves as the identity in this group, and the inverse of Ng in the quotient group is (gN)⁻¹ = (g⁻¹)N.

•	R	U
R	R	U
U	U	R
Group table of the quotient group D4 / R.

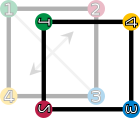
The elements of the quotient group D₄ / R are R itself, which represents the identity, and U = f_vR. The group operation on the quotient is shown at the right. For example, U • U = f_vR • f_vR = (f_v • f_v)R = R. Both the subgroup R = {id, r₁, r₂, r₃}, as well as the corresponding quotient are abelian, whereas D₄ is not abelian. Building bigger groups by smaller ones, such as D₄ from its subgroup R and the quotient D₄ / R is abstracted by a notion called semidirect product.

Quotient and subgroups together form a way of describing every group by its presentation: any group is the quotient of the free group over the generators of the group, quotiented by the subgroup of relations. The dihedral group D₄, for example, can be generated by two elements r and f (for example, r = r₁, the right rotation and f = f_v the vertical (or any other) flip), which means that every symmetry of the square is a finite composition of these two symmetries or their inverses. Together with the relations

r ⁴ = f ² = (rf)² = 1,

the group is completely described. A presentation of a group can also be used to construct the Cayley graph, a device used to graphically capture discrete groups.

Sub- and quotient groups are related in the following way: a subset H of G can be seen as an injective map H → G, i.e. any element of the target has at most one element that maps to it. The counterpart to injective maps are surjective maps (every element of the target is mapped onto), such as the canonical map G → G / N. Interpreting subgroup and quotients in light of these homomorphisms emphasizes the structural concept inherent to these definitions alluded to in the introduction. In general, homomorphisms are neither injective nor surjective. Kernel and image of group homomorphisms and the first isomorphism theorem address this phenomenon.

Examples and applications

A periodic wallpaper gives rise to a wallpaper group.

The fundamental group of a plane minus a point (bold) consists of loops in this area.

Examples and applications of groups abound. A starting point is the group Z of integers with addition as group operation, introduced above. If instead of addition multiplication is considered, one obtains multiplicative groups. These groups are predecessors of important constructions in abstract algebra.

Groups are also applied in many other mathematical areas. Mathematical objects are often examined by associating groups to them and studying the properties of the corresponding groups. For example, Henri Poincaré founded what is now called algebraic topology by introducing the fundamental group. By means of this connection, topological properties such as proximity and continuity translate into properties of groups. For example, the image at the right depicts elements of the fundamental group of a plane minus a point. Elements of the group are given by loops in this area. The blue loop is considered null-homotopic (and thus irrelevant), because it can be shrunk to a point. The presence of the hole prevents the orange loop from being shrunk. The fundamental group of the plane with a point deleted turns out to be infinite cyclic, generated by the orange loop (or any other loop winding once around the hole). This way, the fundamental group detects the hole.

In more recent applications, the influence has also been reversed to motivate geometric constructions by a group-theoretical background. In a similar vein, geometric group theory employs geometric concepts, for example in the study of hyperbolic groups. Further branches crucially applying groups include algebraic geometry and number theory.

In addition to the above theoretical applications, many practical applications of groups exist. Cryptography relies on the combination of the abstract group theory approach together with algorithmical knowledge obtained in computational group theory, in particular when implemented for finite groups. Applications of group theory are not restricted to mathematics; sciences such as physics, chemistry and computer science benefit from the concept.

Numbers

Many number systems, such as the integers and the rationals enjoy a naturally given group structure. In some cases, such as with the rationals, both addition and multiplication operations give rise to group structures. Such number systems are predecessors to more general algebraic structures known as rings and fields.

Integers

The group of integers Z under addition, denoted (Z, +), has been described above. The integers, with the operation of multiplication instead of addition, (Z, ·) do not form a group. The closure, associativity and identity axioms are satisfied, but inverses do not exist: for example, a = 2 is an integer, but the only solution to the equation a · b = 1 in this case is b = 1/2, which is a rational number, but not an integer. Hence not every element of Z has a (multiplicative) inverse.

Rationals

The desire for the existence of multiplicative inverses suggests considering fractions

${\frac {a}{b}}$.

Fractions of integers (with b nonzero) are known as rational numbers. The set of all such fractions is commonly denoted Q. There is still a minor obstacle for (Q, ·), the rationals with multiplication, being a group: because the rational number 0 does not have a multiplicative inverse (i.e., there is no x such that x · 0 = 1), (Q, ·) is still not a group.

However, the set of all nonzero rational numbers Q \ {0} = {q ∈ Q, q ≠ 0} does form an abelian group under multiplication, denoted (Q \ {0}, ·). Associativity and identity element axioms follow from the properties of integers. The closure requirement still holds true after removing zero, because the product of two nonzero rationals is never zero. Finally, the inverse of a/b is b/a, therefore the axiom of the inverse element is satisfied.

The rational numbers (including 0) also form a group under addition. Intertwining addition and multiplication operations yields more complicated structures called rings and—if division is possible, such as in Q—fields, which occupy a central position in abstract algebra. Group theoretic arguments therefore underlie parts of the theory of those entities.

Nonzero integers modulo a prime

For any prime number p, modular arithmetic furnishes the multiplicative group of integers modulo p. Its elements are integers not divisible by p, considered modulo p, i.e. two numbers are considered equivalent if their difference is divisible by p. For example, if p = 5, there are exactly four group elements 1, 2, 3, 4: multiples of 5 are excluded and 6 and −4 are both equivalent to 1 etc. The group operation is given by multiplication. Therefore, 4 · 4 = 1, because the usual product 16 is equivalent to 1, for 5 divides 16 − 1 = 15, denoted

16 ≡ 1 (mod 5).

The primality of p ensures that the product of two integers neither of which is divisible by p is not divisible by p either, hence the indicated set of classes is closed under multiplication. The identity element is 1, as usual for a multiplicative group, and the associativity follows from the corresponding property of integers. Finally, the inverse element axiom requires that given an integer a not divisible by p, there exists an integer b such that

a · b ≡ 1 (mod p), i.e. p divides the difference a · b − 1.

The inverse b can be found by using Bézout's identity and the fact that the greatest common divisor gcd(a, p) equals 1. In the case p = 5 above, the inverse of 4 is 4, and the inverse of 3 is 2, as 3 · 2 = 6 ≡ 1 (mod 5). Hence all group axioms are fulfilled. Actually, this example is similar to (Q\{0}, ·) above, because it turns out to be the multiplicative group of nonzero elements in the finite field F_p, denoted F_p^×. These groups are crucial to public-key cryptography.

Cyclic groups

The 6th complex roots of unity form a cyclic group. z is a primitive element, but z² is not, because the odd powers of z are not a power of z².

A cyclic group is a group all of whose elements are powers (when the group operation is written additively, the term 'multiple' can be used) of a particular element a. In multiplicative notation, the elements of the group are:

..., a⁻³, a⁻², a⁻¹, a⁰ = e, a, a², a³, ...,

where a² means a • a, and a⁻³ stands for a⁻¹ • a⁻¹ • a⁻¹=(a • a • a)⁻¹ etc. Such an element a is called a generator or a primitive element of the group.

A typical example for this class of groups is the group of n-th complex roots of unity, given by complex numbers z satisfying zⁿ = 1 (and whose operation is multiplication). Any cyclic group with n elements is isomorphic to this group. Using some field theory, the group F_p^× can be shown to be cyclic: for p = 5, 3 is a generator since 3¹ = 3, 3² = 9 ≡ 4, 3³ ≡ 2, and 3⁴ ≡ 1. An infinite cyclic group is isomorphic to (Z, +), the group of integers under addition introduced above. As these two prototypes are both abelian, so is any cyclic group.

The study of abelian groups is quite mature, including the fundamental theorem of finitely generated abelian groups; and reflecting this state of affairs, many group-related notions, such as center and commutator, describe the extent to which a given group is not abelian.

Symmetry groups

Modèle:Seealso Symmetry groups are groups consisting of symmetries of given mathematical objects—be they of geometric nature, such as the introductory symmetry group of the square, or of algebraic nature, such as polynomial equations and their solutions. Conceptually, group theory can be thought of as the study of symmetry. Symmetries in mathematics greatly simplify the study of geometrical or analytical objects. A group is said to act on another mathematical object X if every group element performs some operation on X compatibly to the group law. In the rightmost example below, an element of order 7 of the (2,3,7) triangle group acts on the tiling by permuting the highlighted warped triangles (and the other ones, too). By a group action, the group pattern is connected to the structure of the object being acted on.

In chemical fields, such as crystallography, space groups and point groups describe molecular symmetries and crystal symmetries. These symmetries underlie the chemical and physical behavior of these systems, and group theory enables simplification of quantum mechanical analysis of these properties. For example, group theory is used to show that optical transitions between certain quantum levels cannot occur simply because of the symmetry of the states involved.

Not only are groups useful to assess the implications of symmetries in molecules, but surprisingly they also predict that molecules sometimes can change symmetry. The Jahn-Teller effect is a distortion of a molecule of high symmetry when it adopts a particular ground state of lower symmetry from a set of possible ground states that are related to each other by the symmetry operations of the molecule.

Likewise, group theory helps predict the changes in physical properties that occur when a material undergoes a phase transition, for example, from a cubic to a tetrahedral crystalline form. An example is ferroelectric materials, where the change from a paraelectric to a ferroelectric state occurs at the Curie temperature and is related to a change from the high-symmetry paraelectric state to the lower symmetry ferroelectic state, accompanied by a so-called soft phonon mode, a vibrational lattice mode that goes to zero frequency at the transition.

Such spontaneous symmetry breaking has found further application in elementary particle physics, where its occurrence is related to the appearance of Goldstone bosons.

Buckminsterfullerene displays icosahedral symmetry.	Ammonia, NH3. Its symmetry group is of order 6, generated by a 120° rotation and a reflection.	Cubane C8H8 features octahedral symmetry.	Hexaaquacopper(II) complex ion, [Cu(OH2)6]2+. Compared to a perfectly symmetrical shape, the molecule is vertically dilated by about 22% (Jahn-Teller effect).	The (2,3,7) triangle group, a hyperbolic group, acts on this tiling of the hyperbolic plane.

Finite symmetry groups such as the Mathieu groups are used in coding theory, which is in turn applied in error correction of transmitted data, and in CD players. Another application is differential Galois theory, which characterizes functions having antiderivatives of a prescribed form, giving group-theoretic criteria for when solutions of certain differential equations are well-behaved. Geometric properties that remain stable under group actions are investigated in (geometric) invariant theory.

General linear group and representation theory

Two vectors (the left illustration) multiplied by matrices (the middle and right illustrations). The middle illustration represents a clockwise rotation by 90°, while the right-most one stretches the x-coordinate by factor 2.

Matrix groups consist of matrices together with matrix multiplication. The general linear group GL(n, R) consists of all invertible n-by-n matrices with real entries. Its subgroups are referred to as matrix groups or linear groups. The dihedral group example mentioned above can be viewed as a (very small) matrix group. Another important matrix group is the special orthogonal group SO(n). It describes all possible rotations in n dimensions. Via Euler angles, rotation matrices are used in computer graphics.

Representation theory is both an application of the group concept and important for a deeper understanding of groups. It studies the group by its group actions on other spaces. A broad class of group representations are linear representations, i.e. the group is acting on a vector space, such as the three-dimensional Euclidean space R³. A representation of G on an n-dimensional real vector space is simply a group homomorphism

ρ: G → GL(n, R)

from the group to the general linear group. This way, the group operation, which may be abstractly given, translates to the multiplication of matrices making it accessible to explicit computations.

Given a group action, this gives further means to study the object being acted on. On the other hand, it also yields information about the group. Group representations are an organizing principle in the theory of finite groups, Lie groups, algebraic groups and topological groups, especially (locally) compact groups.

Galois groups

Galois groups have been developed to help solving polynomial equations by capturing their symmetry features. For example, the solutions of the quadratic equation ax² + bx + c = 0 are given by

$x={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}.$

Exchanging "+" and "−" in the expression, i.e. permuting the two solutions of the equation can be viewed as a (very simple) group operation. Similar formulae are known for cubic and quartic equations, but do not exist in general for degree 5 and higher. Abstract properties of Galois groups (in particular their solvability) associated to polynomials give a criterion which polynomials do have all their solutions expressible by radicals, i.e. solutions expressible using solely addition, multiplication, and roots similar to the formula above.

The problem can be dealt with even more neatly using field theory: considering the splitting field of a polynomial shifts the problem into the realm of field theory. Modern Galois theory generalizes the above type of Galois groups to field extensions and establishes—via the fundamental theorem of Galois theory—a precise relationship between fields and groups, underlining once again the ubiquity of groups in mathematics.

Finite groups

A group is called finite if it has a finite number of elements. The number of elements is called the order of the group G. An important class is the symmetric groups S_N, the groups of permutations of N letters. For example, the symmetric group on 3 letters S₃ is the group consisting of all possible swaps of the three letters ABC, i.e. contains the elements ABC, ACB, ..., up to CBA, in total 6 (or 3 factorial) elements. This class is fundamental insofar as any finite group can be expressed as a subgroup of a symmetric group S_N for a suitable integer N (Cayley's theorem). Parallel to the group of symmetries of the square above, S₃ can also be interpreted as the group of symmetries of an equilateral triangle.

The order of an element a in a group G is the least positive integer n such that aⁿ = e, where aⁿ represents $\underbrace {a\cdot \ldots \cdot a} _{n}$, i.e. application of the operation • to n copies of a. (If • represents multiplication, then aⁿ corresponds to the n^th power of a.) In infinite groups, such an n may not exist, in which case the order of a is said to be infinity. The order of an element equals the order of the cyclic subgroup generated by this element.

More sophisticated counting techniques, for example counting cosets, yield more precise statements about finite groups: Lagrange's Theorem states that for a finite group G the order of any finite subgroup H divides the order of G. The Sylow theorems give a partial converse.

The dihedral group (discussed above) is a finite group of order 8. The order of r₁ is 4, as is the order of the subgroup R it generates (see above). The order of the reflection elements f_v etc. is 2. Both orders divide 8, as predicted by Lagrange's Theorem. The groups F_p^× above have order p − 1.

Classification of finite simple groups

Mathematicians often strive for a complete classification (or list) of a mathematical notion. In the context of finite groups, this aim quickly leads to difficult and profound mathematics. According to Lagrange's theorem, finite groups of order p, a prime number, are necessarily cyclic (abelian) groups Z_p. Groups of order p² can also be shown to be abelian, a statement which does not generalize to order p³, as the non-abelian group D₄ of order 8 = 2³ above shows. Computer algebra systems can be used to list small groups, but there is no classification of all finite groups. An intermediate step is the classification of finite simple groups. A nontrivial group is called simple if its only normal subgroups are the trivial group and the group itself. The Jordan-Hölder theorem exhibits simple groups as the building blocks for all finite groups. Listing all finite simple groups was a major achievement in contemporary group theory. 1998 Fields Medal winner Richard Borcherds succeeded to prove the monstrous moonshine conjectures, a surprising and deep relation of the largest finite simple sporadic group—the "monster group"—with certain modular functions, a piece of classical complex analysis, and string theory, a theory supposed to unify the description of many physical phenomena.

Groups with additional structure

Many groups are simultaneously groups and examples of other mathematical structures. In the language of category theory, they are group objects in a category, meaning that they are objects (that is, examples of another mathematical structure) which come with transformations (called morphisms) that mimic the group axioms. For example, every group (as defined above) is also a set, so a group is a group object in the category of sets.

Topological groups

The unit circle in the complex plane under complex multiplication is a Lie group and, therefore, a topological group. It is topological since complex multiplication and division are continuous. It is a manifold and thus a Lie group, because every small piece, such as the red arc in the figure, looks like a part of the real line (shown at the bottom).

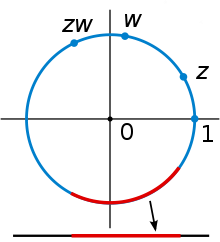
Some topological spaces may be endowed with a group law. In order for the group law and the topology to interweave well, the group operations must be continuous functions, that is, g • h, and g⁻¹ must not vary wildly if g and h vary only little. Such groups are called topological groups, and they are the group objects in the category of topological spaces. The most basic examples are the reals R under addition, (R \ {0}, ·), and similarly with any other topological field such as the complex numbers or p-adic numbers. All of these groups are locally compact, so they have Haar measures and can be studied via harmonic analysis. The former offer an abstract formalism of invariant integrals. Invariance means, in the case of real numbers for example:

$\int f(x)\,dx=\int f(x+c)\,dx$

for any constant c. Matrix groups over these fields fall under this regime, as do adele rings and adelic algebraic groups, which are basic to number theory. Galois groups of infinite field extensions such as the absolute Galois group can also be equipped with a topology, the so-called Krull topology, which in turn is central to generalize the above sketched connection of fields and groups to infinite field extensions. An advanced generalization of this idea, adapted to the needs of algebraic geometry, is the étale fundamental group.

Lie groups

Lie groups (in honor of Sophus Lie) are groups which also have a manifold structure, i.e. they are spaces looking locally like some Euclidean space of the appropriate dimension. Again, the additional structure, here the manifold structure, has to be compatible, i.e. the maps corresponding to multiplication and the inverse have to be smooth.

---

A standard example is the general linear group introduced above: it is an open subset of the space of all n-by-n matrices, because it is given by the inequality

det (A) ≠ 0,

where A denotes an n-by-n matrix.

Lie groups are of fundamental importance in physics: Noether's theorem links continuous symmetries to conserved quantities. Rotation, as well as translations in space and time are basic symmetries of the laws of mechanics. They can, for instance, be used to construct simple models—imposing, say, axial symmetry on a situation will typically lead to significant simplification in the equations one needs to solve to provide a physical description. Another example are the Lorentz transformations, which relate measurements of time and velocity of two observers in motion relative to each other. They can be deduced in a purely group-theoretical way, by expressing the transformations as a rotational symmetry of Minkowski space. The latter serves—in the absence of significant gravitation—as a model of space time in special relativity. The full symmetry group of Minkowski space, i.e. including translations, is known as the Poincaré group. By the above, it plays a pivotal role in special relativity and, by implication, for quantum field theories. Symmetries that vary with location are central to the modern description of physical interactions with the help of gauge theory.

Generalizations

Modèle:Group-like structures In abstract algebra, more general structures are defined by relaxing some of the axioms defining a group. For example, if the requirement that every element has an inverse is eliminated, the resulting algebraic structure is called a monoid. The natural numbers N (including 0) under addition form a monoid, as do the nonzero integers under multiplication (Z \ {0}, ·), see above. There is a general method to formally add inverses to elements to any (abelian) monoid, much the same way as (Q \ {0}, ·) is derived from (Z \ {0}, ·), known as the Grothendieck group. Groupoids are similar to groups except that the composition a • b need not be defined for all a and b. They arise in the study of more complicated forms of symmetry, often in topological and analytical structures, such as the fundamental groupoid. The table gives a list of several structures generalizing groups.

See also

• Group ring
• Group algebra
• Euclidean group
• Grothendieck group
• Symmetry in physics
• CPT symmetry

Notes

---

^ a: Mathematical Reviews lists 3,224 research papers on group theory and its generalizations written in 2005.
^ b: The closure axiom is already implied by the condition that • be a binary operation. Some authors therefore omit this axiom. Modèle:Harvard citations
^ c: See, for example, the books of Lang (2002, 2005) and Herstein (1996, 1975).
^ d: However, a group is not determined by its lattice of subgroups. See Modèle:Harvard citations.
^ e: The fact that the group operation extends this canonically is an instance of a universal property.
^ f: For example, if G is finite, then the size of any subgroup and any quotient group divides the size of G, according to Lagrange's theorem.
^ g: The word homomorphism derives from Greek ὁμός—the same and μορφή—structure.
^ h: The additive notation for elements of a cyclic group would be t • a, t in Z.
^ i: See the Seifert–van Kampen theorem for an example.
^ j: An example is group cohomology of a group which equals the singular homology of its classifying space.
^ k: Elements which do have multiplicative inverses are called units, see Modèle:Harvard citations.
^ l: The transition from the integers to the rationals by adding fractions is generalized by the quotient field.
^ m: The same is true for any field F instead of Q. See Modèle:Harvard citations.
^ n: For example, a finite subgroup of the multiplicative group of a field is necessarily cyclic. See Modèle:Harvard citations. The notions of torsion of a module and simple algebras are other instances of this principle.
^ o: The stated property is a possible definition of prime numbers. See prime element.
^ p: For example, the Diffie-Hellman protocol uses the discrete logarithm.
^ q: The groups of order at most 2000 are known. Up to isomorphism, there are about 49 billion. See Modèle:Harvard citations.
^ r: The gap between the classification of simple groups and the one of all groups lies in the extension problem, a problem too hard to be solved in general. See Modèle:Harvard citations.
^ s: Equivalently, a nontrivial group is simple if its only quotient groups are the trivial group and the group itself. See Modèle:Harvard citations, Modèle:Harvard citations.
^ t: More rigorously, every group is the symmetry group of some graph, see Modèle:Harvard citations.
^ u: More precisely, the monodromy action on the vector space of solutions of the differential equations is considered. See Modèle:Harvard citations.
^ v: See Schwarzschild metric for an example where symmetry greatly reduces the complexity of physical systems.
^ w: This was crucial to the classification of finite simple groups, for example. See Modèle:Harvard citations.
^ x: See, for example, Schur's Lemma for the impact of a group action on simple modules. A more involved example is the action of an absolute Galois group on étale cohomology.
^ y: Injective and surjective maps correspond to mono- and epimorphisms, respectively. They are interchanged when passing to the dual category.

References

General references

• « {{{1}}} », Chapter 2 contains an undergraduate-level exposition of the notions covered in this article.
• « {{{1}}} », Chapter 5 provides a layman-accessible explanation of groups.
• (en) William Fulton et Joe Harris, Representation Theory : A First Course [détail des éditions].
• « {{{1}}} », an elementary introduction.
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».

Special references

• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• (fr) « {{{1}}} ».
• « {{{1}}} ».
• (de) « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} »
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} »
• « {{{1}}} ».
• « {{{1}}} ».
• Modèle:Neukirch ANT.
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} »
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».
• « {{{1}}} ».

Historical references

• « {{{1}}} »
• « {{{1}}} ».
• « {{{1}}} ».
• (de) « {{{1}}} ».
• (fr) « {{{1}}} » (Galois work was first published by Joseph Liouville in 1843).
• (fr) « {{{1}}} ».
• « {{{1}}} ».
• (de) « {{{1}}} ».
• « {{{1}}} »
• « {{{1}}} ».
• « {{{1}}} ».